Warped: Unusual Geometries in Spacetime
Lecture Notes from the 116th Diogenesis Lecture on 2022/7/24 • Read time 17min
We will be going over what the title means, neat things that are possible because of
it (like warp bubbles), and what we could do with it if we can actually achieve a
better understanding of it. Things that we could do with it that would not be so
immediately useful would be things like, nuclear fission and nuclear fusion without
energy loss and special photo/optical systems. More interesting things would be FTL
comms, warp drives, artificial gravity, and artificial wormholes.
So to start, there are different kinds of spaces in mathematics and physicists have
gone through different assessments as to which kind of space we actually have. To
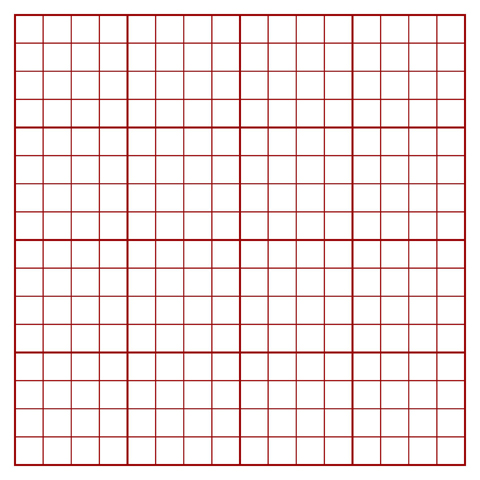
explain this I want you to look at this Cartesian plane.
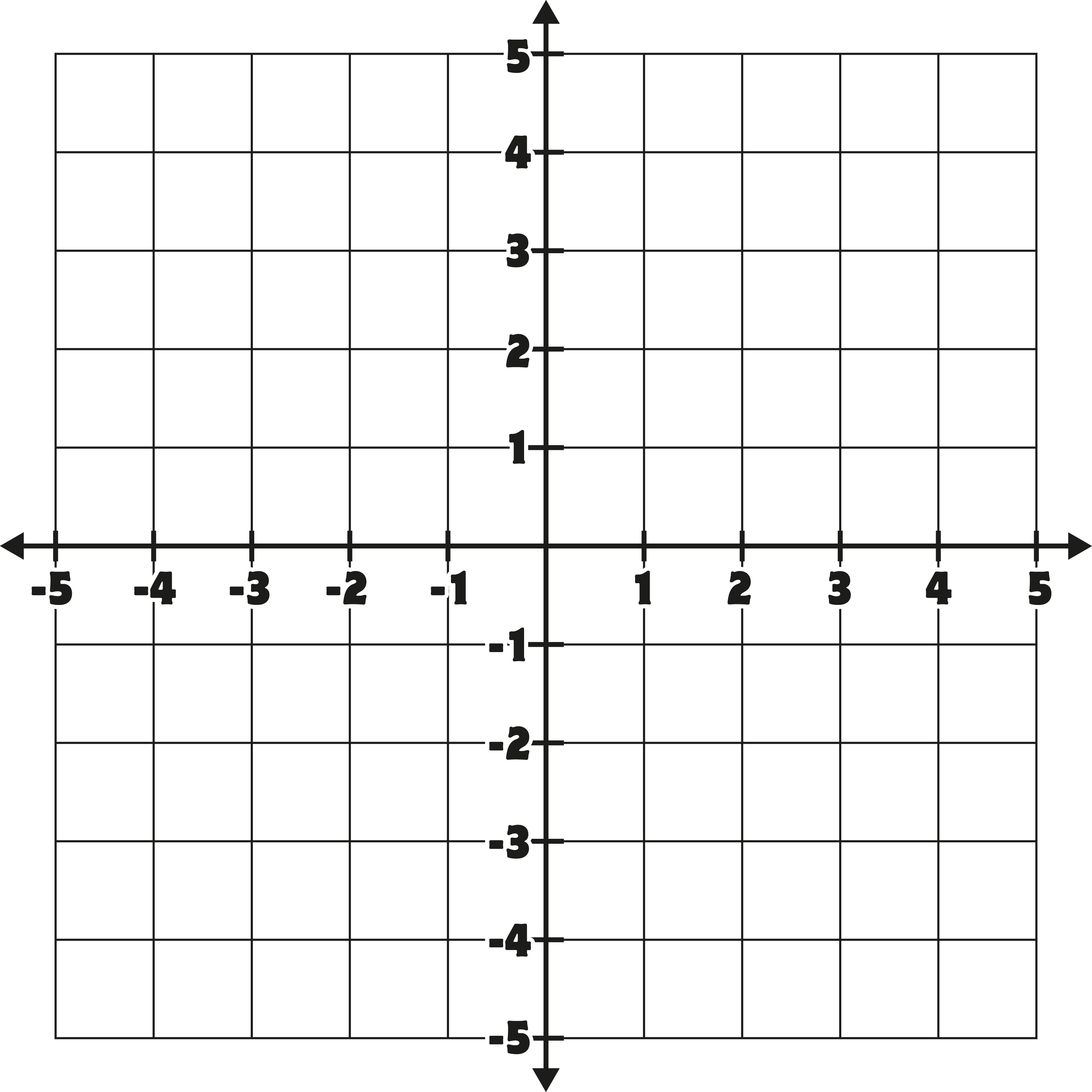 Every intersection of integer lines on a Cartesian plane are equidistant from each
other, meaning all the points you see in that image are one unit away from four other
points. The squares are uniform, there is a regular distance between the entities in
this space, and that regularity is what we call Euclidean. If you add an axis
to the Cartesian plane to make it three-dimensional like this image
Every intersection of integer lines on a Cartesian plane are equidistant from each
other, meaning all the points you see in that image are one unit away from four other
points. The squares are uniform, there is a regular distance between the entities in
this space, and that regularity is what we call Euclidean. If you add an axis
to the Cartesian plane to make it three-dimensional like this image
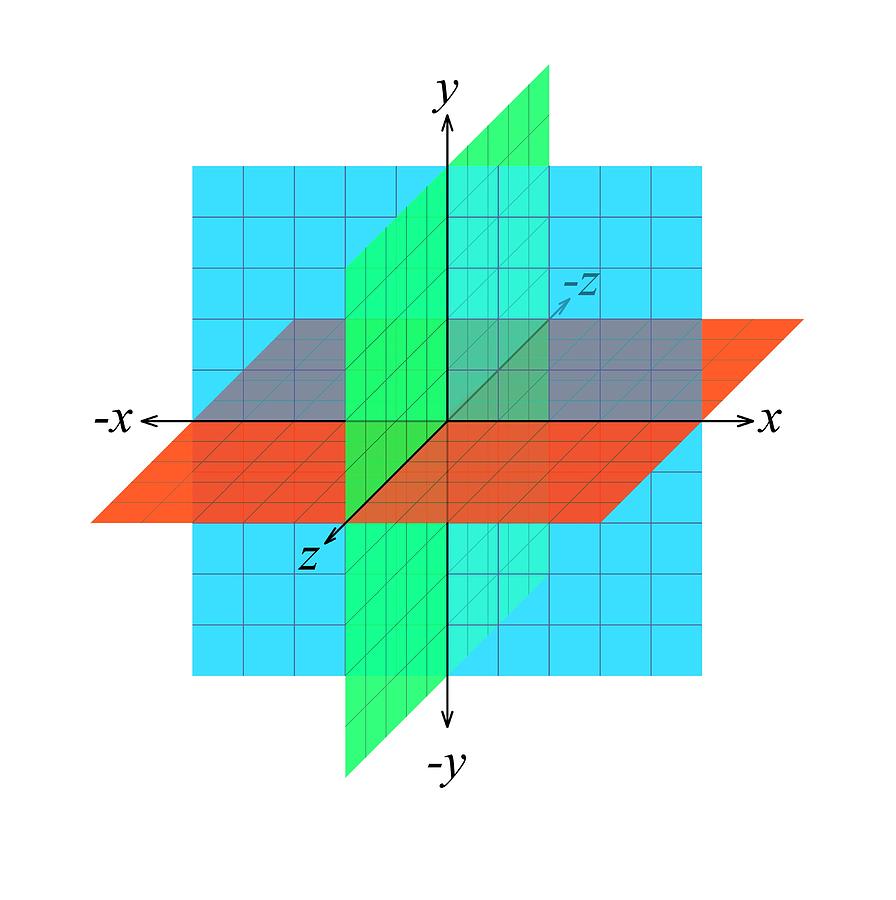 then you have what is called 3D Euclidean space, a space with three dimensions in which
all properties are proportional and regularly spaced.
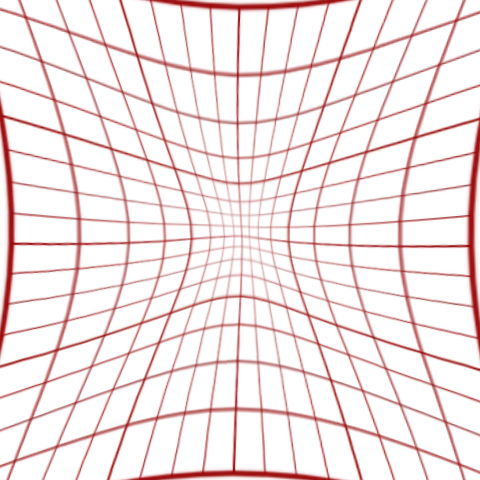
But we can imagine spaces that are irregular in their properties, for example like
this image
then you have what is called 3D Euclidean space, a space with three dimensions in which
all properties are proportional and regularly spaced.
But we can imagine spaces that are irregular in their properties, for example like
this image
 and this image
and this image
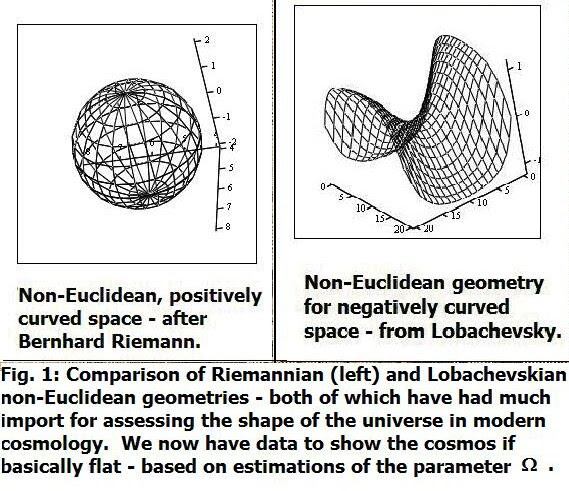 which shows spaces that are bent or folded around themselves. Some of the grid
intersection points in these spaces are really close together and some are really far
away. These are called "Non-Euclidean" spaces and have different properties both in
dimensional capacities and observed topological behavior (topology is the study of
objects whose surfaces can be deformed without destroying the object or surface).
For this lecture I just want you to be aware that there are many different kinds
which shows spaces that are bent or folded around themselves. Some of the grid
intersection points in these spaces are really close together and some are really far
away. These are called "Non-Euclidean" spaces and have different properties both in
dimensional capacities and observed topological behavior (topology is the study of
objects whose surfaces can be deformed without destroying the object or surface).
For this lecture I just want you to be aware that there are many different kinds
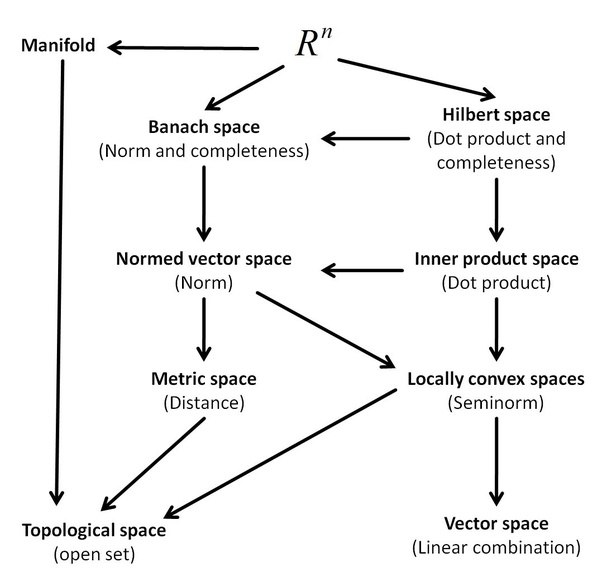 of spaces that mathematicians and physicists look at when working on problems, from
Euclidean spaces to Newtonian spaces, Einsteinean spaces, and others. As to what kind
our universe actually has, physicists have determined we have what is called Hilbert
space,
of spaces that mathematicians and physicists look at when working on problems, from
Euclidean spaces to Newtonian spaces, Einsteinean spaces, and others. As to what kind
our universe actually has, physicists have determined we have what is called Hilbert
space,
 and all you need to know for now is that Hilbert space allows us to combine
potentially infinitely many dimensions into a consistent space like this example
and all you need to know for now is that Hilbert space allows us to combine
potentially infinitely many dimensions into a consistent space like this example
 where three two-dimensional objects are represented as a single six-dimensional object
in only two-dimensions.
Now I realize that this might be confusing, but to mathematicians dimensionality is
somewhat arbitrary. A dimension is not some scifi concept where aliens live, a
dimension is just some axis, vector, array, or set of things you can plot. The formal
definition is that a dimension is the minimum number of coordinates needed to specify
any point within it. For example, a line has a dimension of one because only one
coordinate is needed to specify a point on it.
The only meaningful distinction mathematicians make between 2D and 3D is that 3D has
one more axis than 2D does. Similarly an 80-D grid would just have 77 more axes' than
a 3D grid. So physicists look at this and say that space is whatever number of axes',
or whatever number of dimensions, is appropriate to describe the complete list of
properties that we observe particles actually having. Right now they've settled for
around 12 dimensions.
Keep in mind that the kind of space we have is also non-Euclidean, meaning it can be
bent, stretched, wrapped around itself into potentially closed shapes, and so on. This
is where a lot of work in String theory (or what is called M-Theory) starts - looking
at really small things below the scope of quantum physics.
But for this lecture we will not be looking at small things, we will be looking at the
largest thing - our universe. And so there is an open question in cosmology right now
as to what kind of topology our universe has, meaning what kind of shape the space of
our universe has. As it turns out, that kind of question can actually be answered,
which is pretty crazy.
Of all the Millennium Prize Problems, the only one that's been solved is the Poincaré
conjecture. The premise of the Poincaré conjecture was the question - could we
understand the shape of the space we live in? What's its geometry? What's its
topology? Meaning, is our universe inside a big sphere? A torus? Does it have any kind
of curvature at all or is it a flat plane, etc? And further, can we figure this out if
we exist in higher dimensions? As it turns out, since the Poincaré conjecture has been
proven, we can in principle determine the kind of space we occupy. Maybe not in
practice, but in principle it's solvable.
By most estimations, our universe inhabits a flat plane, that's its shape, which means
it is not a closed or finite universe. But this is all still in Hilbert space with
non-Euclidean properties, and since we now know, or at least have good approximations
of what our universe is like, that knowledge has given us great insight into what we
can do with the space with have. With that done, we can start on the real lecture
content.
With non-Euclidean space, we can take this and stretch it into this, or this,
where three two-dimensional objects are represented as a single six-dimensional object
in only two-dimensions.
Now I realize that this might be confusing, but to mathematicians dimensionality is
somewhat arbitrary. A dimension is not some scifi concept where aliens live, a
dimension is just some axis, vector, array, or set of things you can plot. The formal
definition is that a dimension is the minimum number of coordinates needed to specify
any point within it. For example, a line has a dimension of one because only one
coordinate is needed to specify a point on it.
The only meaningful distinction mathematicians make between 2D and 3D is that 3D has
one more axis than 2D does. Similarly an 80-D grid would just have 77 more axes' than
a 3D grid. So physicists look at this and say that space is whatever number of axes',
or whatever number of dimensions, is appropriate to describe the complete list of
properties that we observe particles actually having. Right now they've settled for
around 12 dimensions.
Keep in mind that the kind of space we have is also non-Euclidean, meaning it can be
bent, stretched, wrapped around itself into potentially closed shapes, and so on. This
is where a lot of work in String theory (or what is called M-Theory) starts - looking
at really small things below the scope of quantum physics.
But for this lecture we will not be looking at small things, we will be looking at the
largest thing - our universe. And so there is an open question in cosmology right now
as to what kind of topology our universe has, meaning what kind of shape the space of
our universe has. As it turns out, that kind of question can actually be answered,
which is pretty crazy.
Of all the Millennium Prize Problems, the only one that's been solved is the Poincaré
conjecture. The premise of the Poincaré conjecture was the question - could we
understand the shape of the space we live in? What's its geometry? What's its
topology? Meaning, is our universe inside a big sphere? A torus? Does it have any kind
of curvature at all or is it a flat plane, etc? And further, can we figure this out if
we exist in higher dimensions? As it turns out, since the Poincaré conjecture has been
proven, we can in principle determine the kind of space we occupy. Maybe not in
practice, but in principle it's solvable.
By most estimations, our universe inhabits a flat plane, that's its shape, which means
it is not a closed or finite universe. But this is all still in Hilbert space with
non-Euclidean properties, and since we now know, or at least have good approximations
of what our universe is like, that knowledge has given us great insight into what we
can do with the space with have. With that done, we can start on the real lecture
content.
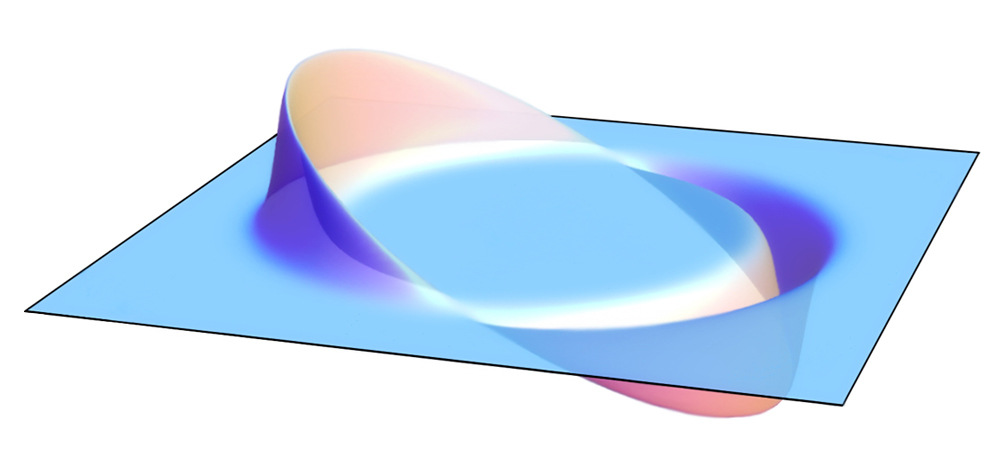
With non-Euclidean space, we can take this and stretch it into this, or this,

 or whatever other configuration we wanted. In fact, that last image is what the effect
of gravity looks like on a 2D plane. This also helps people understand that gravity is
not a force, since it is not a cause but an effect. What we experience as gravity is
just the effect of space being compressed in the direction of mass, pretty similar to
what you see in that last image.
With all this in mind we now get to meet Miguel Alcubierre, who wrote this paper.
This is a famous paper because it showed that while matter cannot move faster than the
speed of light in Einsteinean mechanics, you could still move space faster than the
speed of light, generating famous images that look like this
or whatever other configuration we wanted. In fact, that last image is what the effect
of gravity looks like on a 2D plane. This also helps people understand that gravity is
not a force, since it is not a cause but an effect. What we experience as gravity is
just the effect of space being compressed in the direction of mass, pretty similar to
what you see in that last image.
With all this in mind we now get to meet Miguel Alcubierre, who wrote this paper.
This is a famous paper because it showed that while matter cannot move faster than the
speed of light in Einsteinean mechanics, you could still move space faster than the
speed of light, generating famous images that look like this
 where space is compressed in front of you, wrapped around your sides, and expanded
behind you. However, there are a few problems with Alcubierre's conception of warp.
One is that it requires more energy to warp space than you could fit inside the space
you'd be warping, and also that it would need exotic matter. Another problem was that
there was no way given for how you would get up to that geometry in the image, only
that we knew what it would look like once you were there. This makes warp seem like a
non-starter.
Fortunately it doesn't end there. The beauty of what Alcubierre did wasn't in saying
we could stretch space, we already knew that, the beauty of what he did was in showing
that the way we stretch it is mathematically arbitrary. What I mean by this is that
normally in physics when you want to see the potential effect of something you take
the equation for calculating that thing, put in a range of input values, and see how
the output values that resulted from that equation appear on a graph. So you are
generating a plot on a graph. What Alcubierre did was work backwards - he started with
the graph of values that make the shape you see in that last image and he worked
backwards through the equation to find what input values were required to generate
that graph.
This means we can repeat the same process - we can design any spatial geometry we want
and see how much energy it takes to actually warp space that way. Several people have
been working on this for years, most notably Harold White, who with the combined work
of others, has found that there are several spatial geometries that allow us to warp
space around an object that require less energy than you'd be able to fit in the same
space, solving a problem with Alcubierre's paper. Further, Harold White, through the
work done at the Limitless Space Insititute, has shown that you can exponentially
reduce the required energy again by simply doubling up the effect of the warp field in
a configuration that looks like this.
where space is compressed in front of you, wrapped around your sides, and expanded
behind you. However, there are a few problems with Alcubierre's conception of warp.
One is that it requires more energy to warp space than you could fit inside the space
you'd be warping, and also that it would need exotic matter. Another problem was that
there was no way given for how you would get up to that geometry in the image, only
that we knew what it would look like once you were there. This makes warp seem like a
non-starter.
Fortunately it doesn't end there. The beauty of what Alcubierre did wasn't in saying
we could stretch space, we already knew that, the beauty of what he did was in showing
that the way we stretch it is mathematically arbitrary. What I mean by this is that
normally in physics when you want to see the potential effect of something you take
the equation for calculating that thing, put in a range of input values, and see how
the output values that resulted from that equation appear on a graph. So you are
generating a plot on a graph. What Alcubierre did was work backwards - he started with
the graph of values that make the shape you see in that last image and he worked
backwards through the equation to find what input values were required to generate
that graph.
This means we can repeat the same process - we can design any spatial geometry we want
and see how much energy it takes to actually warp space that way. Several people have
been working on this for years, most notably Harold White, who with the combined work
of others, has found that there are several spatial geometries that allow us to warp
space around an object that require less energy than you'd be able to fit in the same
space, solving a problem with Alcubierre's paper. Further, Harold White, through the
work done at the Limitless Space Insititute, has shown that you can exponentially
reduce the required energy again by simply doubling up the effect of the warp field in
a configuration that looks like this.
 This is not purely theoretical either, as this paper was published in May of last
year demonstrating that a warp bubble was actually observed as a coincidence during an
experiment around similar properties. And better yet a month earlier this paper was
published showing how we could start making warp drives with current technology, the
only catch being that they would run at sub-light speed, but it's a strong start
either way.
The point that I want you all to come away with here is simply that we can and have
warped space in a controllable and reproducible way and there seems to be no
theoretical limit to how much space we can warp at a given time. I personally don't
believe there are causality violations with this since again the matter inside the
bubble isn't violating any Einsteinean mechanics, but this is cutting edge physics and
there are a great number of physicists that still believe faster-than-light warp
shouldn't be possible due to violations of causality. To quickly address them - if FTL
travel isn't possible, then the slower-than-light travel that is possible would lead
to this problem resulting in dilation effects on economics, meaning that instead of
studying physics, they would have much more lucrative careers managing interstellar
finance. Fun fact, that paper was Paul Krugman's graduate thesis. Famous
Nobel-prize-winning economist Paul Krugman was thinking about how to swindle alien
civilizations out of their money before we even had the capacity to visit them. Crazy.
With all that out of the way, we're going to go over what we can actually use this for
now. If you could expand or contract space in a small local area, then you could,
at-will, expand the local space of a group of atoms until the space between their
particles is so wide that the strong nuclear force fails and you get nuclear fission.
Similarly, you could compress the space of some particles so much that they are forced
to fuse together, getting you nuclear fusion the way stars do it. Just the same you
could compress and wrap space around the target area such that all energy inside the
space is directed in one single direction away from the target, which means that yes
this would be a 100% efficient nuclear reactor capable of both fission and fusion.
Further, you could refeed the energy output back into the system and technically have
near-infinite power since you could expand the mass and get the resulting fission
energy, then contract the same mass again, getting the resulting fusion energy, over
and over until the matter simply decoheres.
Just the same, you could use warped space to help bend light in very specific ways as
to focus scanning microscopes that will allow for a level of detail and clarity not
possible otherwise; i.e., you could take detailed 4k images of single atoms or even
individual nuclei.
These are the less interesting applications in my opinion. It's what we do after we
get infinite energy and atomically precise dick pics that interests me. To start, we
could travel faster than the speed of light. This offers huge advantages that will be
obvious to anyone who hears this, but just to make sure we're on the same page, it
means we would now have trivial access to all bodies in our solar system including
trivial transportation of any amount of material we want. No more resource scarcity,
trillion-dollar solid-gold asteroids can now be easily harvested. What's more is that
we aren't limited to our own solar system. We can now travel to other solar systems.
But just how many you may ask? Assuming we never develop a warp drive that goes faster
than ten times the speed of light, that still means that in a single year we could
travel ten lightyears, or 94,607,304,725,808 kilometers, which puts all of these star
star systems
This is not purely theoretical either, as this paper was published in May of last
year demonstrating that a warp bubble was actually observed as a coincidence during an
experiment around similar properties. And better yet a month earlier this paper was
published showing how we could start making warp drives with current technology, the
only catch being that they would run at sub-light speed, but it's a strong start
either way.
The point that I want you all to come away with here is simply that we can and have
warped space in a controllable and reproducible way and there seems to be no
theoretical limit to how much space we can warp at a given time. I personally don't
believe there are causality violations with this since again the matter inside the
bubble isn't violating any Einsteinean mechanics, but this is cutting edge physics and
there are a great number of physicists that still believe faster-than-light warp
shouldn't be possible due to violations of causality. To quickly address them - if FTL
travel isn't possible, then the slower-than-light travel that is possible would lead
to this problem resulting in dilation effects on economics, meaning that instead of
studying physics, they would have much more lucrative careers managing interstellar
finance. Fun fact, that paper was Paul Krugman's graduate thesis. Famous
Nobel-prize-winning economist Paul Krugman was thinking about how to swindle alien
civilizations out of their money before we even had the capacity to visit them. Crazy.
With all that out of the way, we're going to go over what we can actually use this for
now. If you could expand or contract space in a small local area, then you could,
at-will, expand the local space of a group of atoms until the space between their
particles is so wide that the strong nuclear force fails and you get nuclear fission.
Similarly, you could compress the space of some particles so much that they are forced
to fuse together, getting you nuclear fusion the way stars do it. Just the same you
could compress and wrap space around the target area such that all energy inside the
space is directed in one single direction away from the target, which means that yes
this would be a 100% efficient nuclear reactor capable of both fission and fusion.
Further, you could refeed the energy output back into the system and technically have
near-infinite power since you could expand the mass and get the resulting fission
energy, then contract the same mass again, getting the resulting fusion energy, over
and over until the matter simply decoheres.
Just the same, you could use warped space to help bend light in very specific ways as
to focus scanning microscopes that will allow for a level of detail and clarity not
possible otherwise; i.e., you could take detailed 4k images of single atoms or even
individual nuclei.
These are the less interesting applications in my opinion. It's what we do after we
get infinite energy and atomically precise dick pics that interests me. To start, we
could travel faster than the speed of light. This offers huge advantages that will be
obvious to anyone who hears this, but just to make sure we're on the same page, it
means we would now have trivial access to all bodies in our solar system including
trivial transportation of any amount of material we want. No more resource scarcity,
trillion-dollar solid-gold asteroids can now be easily harvested. What's more is that
we aren't limited to our own solar system. We can now travel to other solar systems.
But just how many you may ask? Assuming we never develop a warp drive that goes faster
than ten times the speed of light, that still means that in a single year we could
travel ten lightyears, or 94,607,304,725,808 kilometers, which puts all of these star
star systems
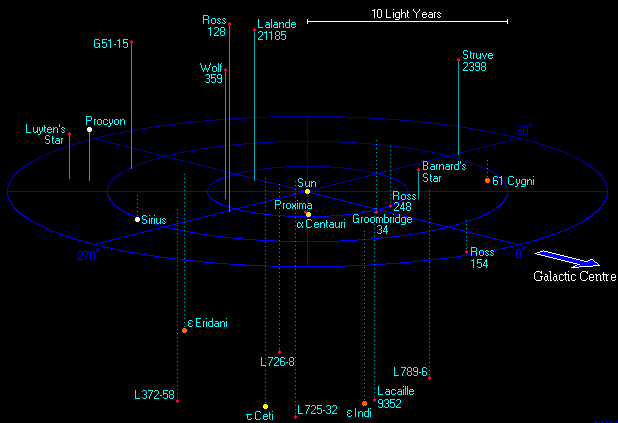 within about a year's travel of us. And we are just getting started.
Flying that FTL ship would be tricky becuase wherever you're looking at before the
flight will be visual data that will become outdated by approximately ten years by the
time you arrive at your destination, but this is where the nascent disciplines of
stellar cartography and stellar navigation get to shine.
Better yet, if we establish faster-than-light communication systems, then you could
course-correct inside of an FTL bubble. I described how this was possible on Inter's
podcast (this paper, this paper, and this), but the short version is via way of
circumventing particle entanglement. Normally you would need to know what information
you're sending in advance in order to observe entanglement between two particles, and
for a long time that meant that physicists thought you could never use entanglement to
communicate new information, but I figured this out on my own and of course actual
researchers figured it out in the paper I just linked.
I borrowed this trick from oldschool information theory back in the days of Bell Labs.
What you do is send a repeating continuous pattern beforehand, kinda like bouncing the
particle back and forth over and over, left, right, left, right, and so on. This
establishes entanglement by way of that pattern, and then you simply look for when you
stop observing entanglement. If you lose entanglement then that means the pattern was
interrupted on the sender's end. An interruption counts as a 1, and observed
entagelement counts as 0. You can now send binary information accross a theoretically
infinite distance. What's even better is that entangment is not just faster than
light, it's perfectly instantaneous. This hurts the heads of phycists and they will
tell you all day and night that what I'm describing is impossible, but I simply refer
you back to the last paper I linked, and you can refer anyone you see disagree with
this to that same paper and let them cry big tears over it.
But again, this is just the start of what's possible. Next on the list is artificial
gravity. I wrote about this before on the /engineering page on snerx in the "Three
insights from an engineering dream" section, but to reiterate, if you have a ship
moving up at 1G of force inside a warp bubble with the warp field moving at 1G of
force in the opposite direction, this creates a uniform 1G of downward force across
all the space inside the warp bubble no matter how big the space is, and there are no
moving parts. Additionally, it would appear to outside observers as if the space
inside was staying still relative to them despite the fact that you are technically
flying upwards inside the bubble. Boom, bam, pow, easy artificial gravity. If you want
to see how this warped artifical gravity differs from mechanical artificial gravity,
you can read the relevant section on the page I just linked.
This brings us to what I think is the most interesting potential technology utilizing
warped space, which is artificial wormholes. These are not like real wormholes, and I
personally don't believe real wormholes could ever exist on a scale larger than a few
atoms wide, but we don't need real wormholes for this to work.
What you see in this image
within about a year's travel of us. And we are just getting started.
Flying that FTL ship would be tricky becuase wherever you're looking at before the
flight will be visual data that will become outdated by approximately ten years by the
time you arrive at your destination, but this is where the nascent disciplines of
stellar cartography and stellar navigation get to shine.
Better yet, if we establish faster-than-light communication systems, then you could
course-correct inside of an FTL bubble. I described how this was possible on Inter's
podcast (this paper, this paper, and this), but the short version is via way of
circumventing particle entanglement. Normally you would need to know what information
you're sending in advance in order to observe entanglement between two particles, and
for a long time that meant that physicists thought you could never use entanglement to
communicate new information, but I figured this out on my own and of course actual
researchers figured it out in the paper I just linked.
I borrowed this trick from oldschool information theory back in the days of Bell Labs.
What you do is send a repeating continuous pattern beforehand, kinda like bouncing the
particle back and forth over and over, left, right, left, right, and so on. This
establishes entanglement by way of that pattern, and then you simply look for when you
stop observing entanglement. If you lose entanglement then that means the pattern was
interrupted on the sender's end. An interruption counts as a 1, and observed
entagelement counts as 0. You can now send binary information accross a theoretically
infinite distance. What's even better is that entangment is not just faster than
light, it's perfectly instantaneous. This hurts the heads of phycists and they will
tell you all day and night that what I'm describing is impossible, but I simply refer
you back to the last paper I linked, and you can refer anyone you see disagree with
this to that same paper and let them cry big tears over it.
But again, this is just the start of what's possible. Next on the list is artificial
gravity. I wrote about this before on the /engineering page on snerx in the "Three
insights from an engineering dream" section, but to reiterate, if you have a ship
moving up at 1G of force inside a warp bubble with the warp field moving at 1G of
force in the opposite direction, this creates a uniform 1G of downward force across
all the space inside the warp bubble no matter how big the space is, and there are no
moving parts. Additionally, it would appear to outside observers as if the space
inside was staying still relative to them despite the fact that you are technically
flying upwards inside the bubble. Boom, bam, pow, easy artificial gravity. If you want
to see how this warped artifical gravity differs from mechanical artificial gravity,
you can read the relevant section on the page I just linked.
This brings us to what I think is the most interesting potential technology utilizing
warped space, which is artificial wormholes. These are not like real wormholes, and I
personally don't believe real wormholes could ever exist on a scale larger than a few
atoms wide, but we don't need real wormholes for this to work.
What you see in this image
 is the graphic I made representing the effect of a warp bubble compressing space in
front of you and expanding it back out behind you, but as you'll see in this image,
is the graphic I made representing the effect of a warp bubble compressing space in
front of you and expanding it back out behind you, but as you'll see in this image,
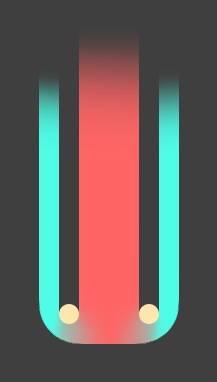 there is a very different way you can achieve a warping effect. If instead of moving
space around an object like a bubble, you move space between two stationary objects,
you then create a gate by which to send ships through faster than the speed of light
despite those ships themselves not having warp drives. This last image is really a 2D
slice of a ring structure but you get the idea.
The way this last graphic works is by expanding the space inside the ring with
preference in one direction, which creates a funnel. You are in a sense making the
space in the funnel 'very thin', allowing things to pass through it easier than
normal. You then send the extra space back around the sides of the funnel, which is a
compressed channel, and is like making that space 'very thick'. I could make a note
here about how just like subjective versus objective time in general relativity there
would also be subjective versus objective space, but for now all you need to know is
that when you approach the mouth of the gate you would get flung many lightyears away.
There are three major benefits this gate, or artifical wormhole, has over a normal
warp drive. The first is that your individual craft doesn't need a functional warp
drive to use the gate. The second is that it's a two-way street, meaning you can come
back from the other side just the same as you went through the entrance. The third is
that this interstellar highway is shielded; the compressed channel around the funnel
stops anything light-speed or slower from penetrating the funnel, since not only would
it take several years to travel through a several-lightyears-thick compressed space,
but that space is also being wrapped around the funnel. So if you can imagine a bridge
with a high arc going over a creek, that's kind of like what the path of a photon
would look like when trying to go through the side of this artificial space funnel.
Even if this gate/artifical wormhole is only able to reach a few light seconds (and
for reference 1 light second is about 299,792km), that would still reduce the normal
nine-months-long trip to Mars down to 187 seconds (~3 minutes) at its shortest
distance 56,000,000km away, and would only take 833 seconds (~14 minutes) at its
furthest distance 225,000,000km away. So this would still be wildly useful even if it
doesn't reach very far or go faster than light.
Everything I just said was an original idea and could be horribly wrong for obvious
reasons that I was far too stupid to realize, but the principles are sound and that's
what matters for this lecture.
Those were a few things we could get from unusual geometries in spacetime. I would
love to hear if you guys come up with more ideas for potential applications of warped
space. This is almost entirely uncharted territory in physics so the sky's the limit.
I also think there's a very open discussion we can have here on what this means for
our capacity to explore and expand into our galaxy, but we already had a lecture on
cosmic sociology (lecture 110 on 2022/5/29) so I'll skip that for today.
there is a very different way you can achieve a warping effect. If instead of moving
space around an object like a bubble, you move space between two stationary objects,
you then create a gate by which to send ships through faster than the speed of light
despite those ships themselves not having warp drives. This last image is really a 2D
slice of a ring structure but you get the idea.
The way this last graphic works is by expanding the space inside the ring with
preference in one direction, which creates a funnel. You are in a sense making the
space in the funnel 'very thin', allowing things to pass through it easier than
normal. You then send the extra space back around the sides of the funnel, which is a
compressed channel, and is like making that space 'very thick'. I could make a note
here about how just like subjective versus objective time in general relativity there
would also be subjective versus objective space, but for now all you need to know is
that when you approach the mouth of the gate you would get flung many lightyears away.
There are three major benefits this gate, or artifical wormhole, has over a normal
warp drive. The first is that your individual craft doesn't need a functional warp
drive to use the gate. The second is that it's a two-way street, meaning you can come
back from the other side just the same as you went through the entrance. The third is
that this interstellar highway is shielded; the compressed channel around the funnel
stops anything light-speed or slower from penetrating the funnel, since not only would
it take several years to travel through a several-lightyears-thick compressed space,
but that space is also being wrapped around the funnel. So if you can imagine a bridge
with a high arc going over a creek, that's kind of like what the path of a photon
would look like when trying to go through the side of this artificial space funnel.
Even if this gate/artifical wormhole is only able to reach a few light seconds (and
for reference 1 light second is about 299,792km), that would still reduce the normal
nine-months-long trip to Mars down to 187 seconds (~3 minutes) at its shortest
distance 56,000,000km away, and would only take 833 seconds (~14 minutes) at its
furthest distance 225,000,000km away. So this would still be wildly useful even if it
doesn't reach very far or go faster than light.
Everything I just said was an original idea and could be horribly wrong for obvious
reasons that I was far too stupid to realize, but the principles are sound and that's
what matters for this lecture.
Those were a few things we could get from unusual geometries in spacetime. I would
love to hear if you guys come up with more ideas for potential applications of warped
space. This is almost entirely uncharted territory in physics so the sky's the limit.
I also think there's a very open discussion we can have here on what this means for
our capacity to explore and expand into our galaxy, but we already had a lecture on
cosmic sociology (lecture 110 on 2022/5/29) so I'll skip that for today.
 Every intersection of integer lines on a Cartesian plane are equidistant from each
other, meaning all the points you see in that image are one unit away from four other
points. The squares are uniform, there is a regular distance between the entities in
this space, and that regularity is what we call Euclidean. If you add an axis
to the Cartesian plane to make it three-dimensional like this image
Every intersection of integer lines on a Cartesian plane are equidistant from each
other, meaning all the points you see in that image are one unit away from four other
points. The squares are uniform, there is a regular distance between the entities in
this space, and that regularity is what we call Euclidean. If you add an axis
to the Cartesian plane to make it three-dimensional like this image
 then you have what is called 3D Euclidean space, a space with three dimensions in which
all properties are proportional and regularly spaced.
But we can imagine spaces that are irregular in their properties, for example like
this image
then you have what is called 3D Euclidean space, a space with three dimensions in which
all properties are proportional and regularly spaced.
But we can imagine spaces that are irregular in their properties, for example like
this image
 and this image
and this image
 which shows spaces that are bent or folded around themselves. Some of the grid
intersection points in these spaces are really close together and some are really far
away. These are called "Non-Euclidean" spaces and have different properties both in
dimensional capacities and observed topological behavior (topology is the study of
objects whose surfaces can be deformed without destroying the object or surface).
For this lecture I just want you to be aware that there are many different kinds
which shows spaces that are bent or folded around themselves. Some of the grid
intersection points in these spaces are really close together and some are really far
away. These are called "Non-Euclidean" spaces and have different properties both in
dimensional capacities and observed topological behavior (topology is the study of
objects whose surfaces can be deformed without destroying the object or surface).
For this lecture I just want you to be aware that there are many different kinds
 of spaces that mathematicians and physicists look at when working on problems, from
Euclidean spaces to Newtonian spaces, Einsteinean spaces, and others. As to what kind
our universe actually has, physicists have determined we have what is called Hilbert
space,
of spaces that mathematicians and physicists look at when working on problems, from
Euclidean spaces to Newtonian spaces, Einsteinean spaces, and others. As to what kind
our universe actually has, physicists have determined we have what is called Hilbert
space,
 and all you need to know for now is that Hilbert space allows us to combine
potentially infinitely many dimensions into a consistent space like this example
and all you need to know for now is that Hilbert space allows us to combine
potentially infinitely many dimensions into a consistent space like this example
 where three two-dimensional objects are represented as a single six-dimensional object
in only two-dimensions.
Now I realize that this might be confusing, but to mathematicians dimensionality is
somewhat arbitrary. A dimension is not some scifi concept where aliens live, a
dimension is just some axis, vector, array, or set of things you can plot. The formal
definition is that a dimension is the minimum number of coordinates needed to specify
any point within it. For example, a line has a dimension of one because only one
coordinate is needed to specify a point on it.
The only meaningful distinction mathematicians make between 2D and 3D is that 3D has
one more axis than 2D does. Similarly an 80-D grid would just have 77 more axes' than
a 3D grid. So physicists look at this and say that space is whatever number of axes',
or whatever number of dimensions, is appropriate to describe the complete list of
properties that we observe particles actually having. Right now they've settled for
around 12 dimensions.
Keep in mind that the kind of space we have is also non-Euclidean, meaning it can be
bent, stretched, wrapped around itself into potentially closed shapes, and so on. This
is where a lot of work in String theory (or what is called M-Theory) starts - looking
at really small things below the scope of quantum physics.
But for this lecture we will not be looking at small things, we will be looking at the
largest thing - our universe. And so there is an open question in cosmology right now
as to what kind of topology our universe has, meaning what kind of shape the space of
our universe has. As it turns out, that kind of question can actually be answered,
which is pretty crazy.
Of all the Millennium Prize Problems, the only one that's been solved is the Poincaré
conjecture. The premise of the Poincaré conjecture was the question - could we
understand the shape of the space we live in? What's its geometry? What's its
topology? Meaning, is our universe inside a big sphere? A torus? Does it have any kind
of curvature at all or is it a flat plane, etc? And further, can we figure this out if
we exist in higher dimensions? As it turns out, since the Poincaré conjecture has been
proven, we can in principle determine the kind of space we occupy. Maybe not in
practice, but in principle it's solvable.
By most estimations, our universe inhabits a flat plane, that's its shape, which means
it is not a closed or finite universe. But this is all still in Hilbert space with
non-Euclidean properties, and since we now know, or at least have good approximations
of what our universe is like, that knowledge has given us great insight into what we
can do with the space with have. With that done, we can start on the real lecture
content.
With non-Euclidean space, we can take this and stretch it into this, or this,
where three two-dimensional objects are represented as a single six-dimensional object
in only two-dimensions.
Now I realize that this might be confusing, but to mathematicians dimensionality is
somewhat arbitrary. A dimension is not some scifi concept where aliens live, a
dimension is just some axis, vector, array, or set of things you can plot. The formal
definition is that a dimension is the minimum number of coordinates needed to specify
any point within it. For example, a line has a dimension of one because only one
coordinate is needed to specify a point on it.
The only meaningful distinction mathematicians make between 2D and 3D is that 3D has
one more axis than 2D does. Similarly an 80-D grid would just have 77 more axes' than
a 3D grid. So physicists look at this and say that space is whatever number of axes',
or whatever number of dimensions, is appropriate to describe the complete list of
properties that we observe particles actually having. Right now they've settled for
around 12 dimensions.
Keep in mind that the kind of space we have is also non-Euclidean, meaning it can be
bent, stretched, wrapped around itself into potentially closed shapes, and so on. This
is where a lot of work in String theory (or what is called M-Theory) starts - looking
at really small things below the scope of quantum physics.
But for this lecture we will not be looking at small things, we will be looking at the
largest thing - our universe. And so there is an open question in cosmology right now
as to what kind of topology our universe has, meaning what kind of shape the space of
our universe has. As it turns out, that kind of question can actually be answered,
which is pretty crazy.
Of all the Millennium Prize Problems, the only one that's been solved is the Poincaré
conjecture. The premise of the Poincaré conjecture was the question - could we
understand the shape of the space we live in? What's its geometry? What's its
topology? Meaning, is our universe inside a big sphere? A torus? Does it have any kind
of curvature at all or is it a flat plane, etc? And further, can we figure this out if
we exist in higher dimensions? As it turns out, since the Poincaré conjecture has been
proven, we can in principle determine the kind of space we occupy. Maybe not in
practice, but in principle it's solvable.
By most estimations, our universe inhabits a flat plane, that's its shape, which means
it is not a closed or finite universe. But this is all still in Hilbert space with
non-Euclidean properties, and since we now know, or at least have good approximations
of what our universe is like, that knowledge has given us great insight into what we
can do with the space with have. With that done, we can start on the real lecture
content.
With non-Euclidean space, we can take this and stretch it into this, or this,


 or whatever other configuration we wanted. In fact, that last image is what the effect
of gravity looks like on a 2D plane. This also helps people understand that gravity is
not a force, since it is not a cause but an effect. What we experience as gravity is
just the effect of space being compressed in the direction of mass, pretty similar to
what you see in that last image.
With all this in mind we now get to meet Miguel Alcubierre, who wrote this paper.
This is a famous paper because it showed that while matter cannot move faster than the
speed of light in Einsteinean mechanics, you could still move space faster than the
speed of light, generating famous images that look like this
or whatever other configuration we wanted. In fact, that last image is what the effect
of gravity looks like on a 2D plane. This also helps people understand that gravity is
not a force, since it is not a cause but an effect. What we experience as gravity is
just the effect of space being compressed in the direction of mass, pretty similar to
what you see in that last image.
With all this in mind we now get to meet Miguel Alcubierre, who wrote this paper.
This is a famous paper because it showed that while matter cannot move faster than the
speed of light in Einsteinean mechanics, you could still move space faster than the
speed of light, generating famous images that look like this
 where space is compressed in front of you, wrapped around your sides, and expanded
behind you. However, there are a few problems with Alcubierre's conception of warp.
One is that it requires more energy to warp space than you could fit inside the space
you'd be warping, and also that it would need exotic matter. Another problem was that
there was no way given for how you would get up to that geometry in the image, only
that we knew what it would look like once you were there. This makes warp seem like a
non-starter.
Fortunately it doesn't end there. The beauty of what Alcubierre did wasn't in saying
we could stretch space, we already knew that, the beauty of what he did was in showing
that the way we stretch it is mathematically arbitrary. What I mean by this is that
normally in physics when you want to see the potential effect of something you take
the equation for calculating that thing, put in a range of input values, and see how
the output values that resulted from that equation appear on a graph. So you are
generating a plot on a graph. What Alcubierre did was work backwards - he started with
the graph of values that make the shape you see in that last image and he worked
backwards through the equation to find what input values were required to generate
that graph.
This means we can repeat the same process - we can design any spatial geometry we want
and see how much energy it takes to actually warp space that way. Several people have
been working on this for years, most notably Harold White, who with the combined work
of others, has found that there are several spatial geometries that allow us to warp
space around an object that require less energy than you'd be able to fit in the same
space, solving a problem with Alcubierre's paper. Further, Harold White, through the
work done at the Limitless Space Insititute, has shown that you can exponentially
reduce the required energy again by simply doubling up the effect of the warp field in
a configuration that looks like this.
where space is compressed in front of you, wrapped around your sides, and expanded
behind you. However, there are a few problems with Alcubierre's conception of warp.
One is that it requires more energy to warp space than you could fit inside the space
you'd be warping, and also that it would need exotic matter. Another problem was that
there was no way given for how you would get up to that geometry in the image, only
that we knew what it would look like once you were there. This makes warp seem like a
non-starter.
Fortunately it doesn't end there. The beauty of what Alcubierre did wasn't in saying
we could stretch space, we already knew that, the beauty of what he did was in showing
that the way we stretch it is mathematically arbitrary. What I mean by this is that
normally in physics when you want to see the potential effect of something you take
the equation for calculating that thing, put in a range of input values, and see how
the output values that resulted from that equation appear on a graph. So you are
generating a plot on a graph. What Alcubierre did was work backwards - he started with
the graph of values that make the shape you see in that last image and he worked
backwards through the equation to find what input values were required to generate
that graph.
This means we can repeat the same process - we can design any spatial geometry we want
and see how much energy it takes to actually warp space that way. Several people have
been working on this for years, most notably Harold White, who with the combined work
of others, has found that there are several spatial geometries that allow us to warp
space around an object that require less energy than you'd be able to fit in the same
space, solving a problem with Alcubierre's paper. Further, Harold White, through the
work done at the Limitless Space Insititute, has shown that you can exponentially
reduce the required energy again by simply doubling up the effect of the warp field in
a configuration that looks like this.
 This is not purely theoretical either, as this paper was published in May of last
year demonstrating that a warp bubble was actually observed as a coincidence during an
experiment around similar properties. And better yet a month earlier this paper was
published showing how we could start making warp drives with current technology, the
only catch being that they would run at sub-light speed, but it's a strong start
either way.
The point that I want you all to come away with here is simply that we can and have
warped space in a controllable and reproducible way and there seems to be no
theoretical limit to how much space we can warp at a given time. I personally don't
believe there are causality violations with this since again the matter inside the
bubble isn't violating any Einsteinean mechanics, but this is cutting edge physics and
there are a great number of physicists that still believe faster-than-light warp
shouldn't be possible due to violations of causality. To quickly address them - if FTL
travel isn't possible, then the slower-than-light travel that is possible would lead
to this problem resulting in dilation effects on economics, meaning that instead of
studying physics, they would have much more lucrative careers managing interstellar
finance. Fun fact, that paper was Paul Krugman's graduate thesis. Famous
Nobel-prize-winning economist Paul Krugman was thinking about how to swindle alien
civilizations out of their money before we even had the capacity to visit them. Crazy.
With all that out of the way, we're going to go over what we can actually use this for
now. If you could expand or contract space in a small local area, then you could,
at-will, expand the local space of a group of atoms until the space between their
particles is so wide that the strong nuclear force fails and you get nuclear fission.
Similarly, you could compress the space of some particles so much that they are forced
to fuse together, getting you nuclear fusion the way stars do it. Just the same you
could compress and wrap space around the target area such that all energy inside the
space is directed in one single direction away from the target, which means that yes
this would be a 100% efficient nuclear reactor capable of both fission and fusion.
Further, you could refeed the energy output back into the system and technically have
near-infinite power since you could expand the mass and get the resulting fission
energy, then contract the same mass again, getting the resulting fusion energy, over
and over until the matter simply decoheres.
Just the same, you could use warped space to help bend light in very specific ways as
to focus scanning microscopes that will allow for a level of detail and clarity not
possible otherwise; i.e., you could take detailed 4k images of single atoms or even
individual nuclei.
These are the less interesting applications in my opinion. It's what we do after we
get infinite energy and atomically precise dick pics that interests me. To start, we
could travel faster than the speed of light. This offers huge advantages that will be
obvious to anyone who hears this, but just to make sure we're on the same page, it
means we would now have trivial access to all bodies in our solar system including
trivial transportation of any amount of material we want. No more resource scarcity,
trillion-dollar solid-gold asteroids can now be easily harvested. What's more is that
we aren't limited to our own solar system. We can now travel to other solar systems.
But just how many you may ask? Assuming we never develop a warp drive that goes faster
than ten times the speed of light, that still means that in a single year we could
travel ten lightyears, or 94,607,304,725,808 kilometers, which puts all of these star
star systems
This is not purely theoretical either, as this paper was published in May of last
year demonstrating that a warp bubble was actually observed as a coincidence during an
experiment around similar properties. And better yet a month earlier this paper was
published showing how we could start making warp drives with current technology, the
only catch being that they would run at sub-light speed, but it's a strong start
either way.
The point that I want you all to come away with here is simply that we can and have
warped space in a controllable and reproducible way and there seems to be no
theoretical limit to how much space we can warp at a given time. I personally don't
believe there are causality violations with this since again the matter inside the
bubble isn't violating any Einsteinean mechanics, but this is cutting edge physics and
there are a great number of physicists that still believe faster-than-light warp
shouldn't be possible due to violations of causality. To quickly address them - if FTL
travel isn't possible, then the slower-than-light travel that is possible would lead
to this problem resulting in dilation effects on economics, meaning that instead of
studying physics, they would have much more lucrative careers managing interstellar
finance. Fun fact, that paper was Paul Krugman's graduate thesis. Famous
Nobel-prize-winning economist Paul Krugman was thinking about how to swindle alien
civilizations out of their money before we even had the capacity to visit them. Crazy.
With all that out of the way, we're going to go over what we can actually use this for
now. If you could expand or contract space in a small local area, then you could,
at-will, expand the local space of a group of atoms until the space between their
particles is so wide that the strong nuclear force fails and you get nuclear fission.
Similarly, you could compress the space of some particles so much that they are forced
to fuse together, getting you nuclear fusion the way stars do it. Just the same you
could compress and wrap space around the target area such that all energy inside the
space is directed in one single direction away from the target, which means that yes
this would be a 100% efficient nuclear reactor capable of both fission and fusion.
Further, you could refeed the energy output back into the system and technically have
near-infinite power since you could expand the mass and get the resulting fission
energy, then contract the same mass again, getting the resulting fusion energy, over
and over until the matter simply decoheres.
Just the same, you could use warped space to help bend light in very specific ways as
to focus scanning microscopes that will allow for a level of detail and clarity not
possible otherwise; i.e., you could take detailed 4k images of single atoms or even
individual nuclei.
These are the less interesting applications in my opinion. It's what we do after we
get infinite energy and atomically precise dick pics that interests me. To start, we
could travel faster than the speed of light. This offers huge advantages that will be
obvious to anyone who hears this, but just to make sure we're on the same page, it
means we would now have trivial access to all bodies in our solar system including
trivial transportation of any amount of material we want. No more resource scarcity,
trillion-dollar solid-gold asteroids can now be easily harvested. What's more is that
we aren't limited to our own solar system. We can now travel to other solar systems.
But just how many you may ask? Assuming we never develop a warp drive that goes faster
than ten times the speed of light, that still means that in a single year we could
travel ten lightyears, or 94,607,304,725,808 kilometers, which puts all of these star
star systems
 within about a year's travel of us. And we are just getting started.
Flying that FTL ship would be tricky becuase wherever you're looking at before the
flight will be visual data that will become outdated by approximately ten years by the
time you arrive at your destination, but this is where the nascent disciplines of
stellar cartography and stellar navigation get to shine.
Better yet, if we establish faster-than-light communication systems, then you could
course-correct inside of an FTL bubble. I described how this was possible on Inter's
podcast (this paper, this paper, and this), but the short version is via way of
circumventing particle entanglement. Normally you would need to know what information
you're sending in advance in order to observe entanglement between two particles, and
for a long time that meant that physicists thought you could never use entanglement to
communicate new information, but I figured this out on my own and of course actual
researchers figured it out in the paper I just linked.
I borrowed this trick from oldschool information theory back in the days of Bell Labs.
What you do is send a repeating continuous pattern beforehand, kinda like bouncing the
particle back and forth over and over, left, right, left, right, and so on. This
establishes entanglement by way of that pattern, and then you simply look for when you
stop observing entanglement. If you lose entanglement then that means the pattern was
interrupted on the sender's end. An interruption counts as a 1, and observed
entagelement counts as 0. You can now send binary information accross a theoretically
infinite distance. What's even better is that entangment is not just faster than
light, it's perfectly instantaneous. This hurts the heads of phycists and they will
tell you all day and night that what I'm describing is impossible, but I simply refer
you back to the last paper I linked, and you can refer anyone you see disagree with
this to that same paper and let them cry big tears over it.
But again, this is just the start of what's possible. Next on the list is artificial
gravity. I wrote about this before on the /engineering page on snerx in the "Three
insights from an engineering dream" section, but to reiterate, if you have a ship
moving up at 1G of force inside a warp bubble with the warp field moving at 1G of
force in the opposite direction, this creates a uniform 1G of downward force across
all the space inside the warp bubble no matter how big the space is, and there are no
moving parts. Additionally, it would appear to outside observers as if the space
inside was staying still relative to them despite the fact that you are technically
flying upwards inside the bubble. Boom, bam, pow, easy artificial gravity. If you want
to see how this warped artifical gravity differs from mechanical artificial gravity,
you can read the relevant section on the page I just linked.
This brings us to what I think is the most interesting potential technology utilizing
warped space, which is artificial wormholes. These are not like real wormholes, and I
personally don't believe real wormholes could ever exist on a scale larger than a few
atoms wide, but we don't need real wormholes for this to work.
What you see in this image
within about a year's travel of us. And we are just getting started.
Flying that FTL ship would be tricky becuase wherever you're looking at before the
flight will be visual data that will become outdated by approximately ten years by the
time you arrive at your destination, but this is where the nascent disciplines of
stellar cartography and stellar navigation get to shine.
Better yet, if we establish faster-than-light communication systems, then you could
course-correct inside of an FTL bubble. I described how this was possible on Inter's
podcast (this paper, this paper, and this), but the short version is via way of
circumventing particle entanglement. Normally you would need to know what information
you're sending in advance in order to observe entanglement between two particles, and
for a long time that meant that physicists thought you could never use entanglement to
communicate new information, but I figured this out on my own and of course actual
researchers figured it out in the paper I just linked.
I borrowed this trick from oldschool information theory back in the days of Bell Labs.
What you do is send a repeating continuous pattern beforehand, kinda like bouncing the
particle back and forth over and over, left, right, left, right, and so on. This
establishes entanglement by way of that pattern, and then you simply look for when you
stop observing entanglement. If you lose entanglement then that means the pattern was
interrupted on the sender's end. An interruption counts as a 1, and observed
entagelement counts as 0. You can now send binary information accross a theoretically
infinite distance. What's even better is that entangment is not just faster than
light, it's perfectly instantaneous. This hurts the heads of phycists and they will
tell you all day and night that what I'm describing is impossible, but I simply refer
you back to the last paper I linked, and you can refer anyone you see disagree with
this to that same paper and let them cry big tears over it.
But again, this is just the start of what's possible. Next on the list is artificial
gravity. I wrote about this before on the /engineering page on snerx in the "Three
insights from an engineering dream" section, but to reiterate, if you have a ship
moving up at 1G of force inside a warp bubble with the warp field moving at 1G of
force in the opposite direction, this creates a uniform 1G of downward force across
all the space inside the warp bubble no matter how big the space is, and there are no
moving parts. Additionally, it would appear to outside observers as if the space
inside was staying still relative to them despite the fact that you are technically
flying upwards inside the bubble. Boom, bam, pow, easy artificial gravity. If you want
to see how this warped artifical gravity differs from mechanical artificial gravity,
you can read the relevant section on the page I just linked.
This brings us to what I think is the most interesting potential technology utilizing
warped space, which is artificial wormholes. These are not like real wormholes, and I
personally don't believe real wormholes could ever exist on a scale larger than a few
atoms wide, but we don't need real wormholes for this to work.
What you see in this image
 is the graphic I made representing the effect of a warp bubble compressing space in
front of you and expanding it back out behind you, but as you'll see in this image,
is the graphic I made representing the effect of a warp bubble compressing space in
front of you and expanding it back out behind you, but as you'll see in this image,
 there is a very different way you can achieve a warping effect. If instead of moving
space around an object like a bubble, you move space between two stationary objects,
you then create a gate by which to send ships through faster than the speed of light
despite those ships themselves not having warp drives. This last image is really a 2D
slice of a ring structure but you get the idea.
The way this last graphic works is by expanding the space inside the ring with
preference in one direction, which creates a funnel. You are in a sense making the
space in the funnel 'very thin', allowing things to pass through it easier than
normal. You then send the extra space back around the sides of the funnel, which is a
compressed channel, and is like making that space 'very thick'. I could make a note
here about how just like subjective versus objective time in general relativity there
would also be subjective versus objective space, but for now all you need to know is
that when you approach the mouth of the gate you would get flung many lightyears away.
There are three major benefits this gate, or artifical wormhole, has over a normal
warp drive. The first is that your individual craft doesn't need a functional warp
drive to use the gate. The second is that it's a two-way street, meaning you can come
back from the other side just the same as you went through the entrance. The third is
that this interstellar highway is shielded; the compressed channel around the funnel
stops anything light-speed or slower from penetrating the funnel, since not only would
it take several years to travel through a several-lightyears-thick compressed space,
but that space is also being wrapped around the funnel. So if you can imagine a bridge
with a high arc going over a creek, that's kind of like what the path of a photon
would look like when trying to go through the side of this artificial space funnel.
Even if this gate/artifical wormhole is only able to reach a few light seconds (and
for reference 1 light second is about 299,792km), that would still reduce the normal
nine-months-long trip to Mars down to 187 seconds (~3 minutes) at its shortest
distance 56,000,000km away, and would only take 833 seconds (~14 minutes) at its
furthest distance 225,000,000km away. So this would still be wildly useful even if it
doesn't reach very far or go faster than light.
Everything I just said was an original idea and could be horribly wrong for obvious
reasons that I was far too stupid to realize, but the principles are sound and that's
what matters for this lecture.
Those were a few things we could get from unusual geometries in spacetime. I would
love to hear if you guys come up with more ideas for potential applications of warped
space. This is almost entirely uncharted territory in physics so the sky's the limit.
I also think there's a very open discussion we can have here on what this means for
our capacity to explore and expand into our galaxy, but we already had a lecture on
cosmic sociology (lecture 110 on 2022/5/29) so I'll skip that for today.
there is a very different way you can achieve a warping effect. If instead of moving
space around an object like a bubble, you move space between two stationary objects,
you then create a gate by which to send ships through faster than the speed of light
despite those ships themselves not having warp drives. This last image is really a 2D
slice of a ring structure but you get the idea.
The way this last graphic works is by expanding the space inside the ring with
preference in one direction, which creates a funnel. You are in a sense making the
space in the funnel 'very thin', allowing things to pass through it easier than
normal. You then send the extra space back around the sides of the funnel, which is a
compressed channel, and is like making that space 'very thick'. I could make a note
here about how just like subjective versus objective time in general relativity there
would also be subjective versus objective space, but for now all you need to know is
that when you approach the mouth of the gate you would get flung many lightyears away.
There are three major benefits this gate, or artifical wormhole, has over a normal
warp drive. The first is that your individual craft doesn't need a functional warp
drive to use the gate. The second is that it's a two-way street, meaning you can come
back from the other side just the same as you went through the entrance. The third is
that this interstellar highway is shielded; the compressed channel around the funnel
stops anything light-speed or slower from penetrating the funnel, since not only would
it take several years to travel through a several-lightyears-thick compressed space,
but that space is also being wrapped around the funnel. So if you can imagine a bridge
with a high arc going over a creek, that's kind of like what the path of a photon
would look like when trying to go through the side of this artificial space funnel.
Even if this gate/artifical wormhole is only able to reach a few light seconds (and
for reference 1 light second is about 299,792km), that would still reduce the normal
nine-months-long trip to Mars down to 187 seconds (~3 minutes) at its shortest
distance 56,000,000km away, and would only take 833 seconds (~14 minutes) at its
furthest distance 225,000,000km away. So this would still be wildly useful even if it
doesn't reach very far or go faster than light.
Everything I just said was an original idea and could be horribly wrong for obvious
reasons that I was far too stupid to realize, but the principles are sound and that's
what matters for this lecture.
Those were a few things we could get from unusual geometries in spacetime. I would
love to hear if you guys come up with more ideas for potential applications of warped
space. This is almost entirely uncharted territory in physics so the sky's the limit.
I also think there's a very open discussion we can have here on what this means for
our capacity to explore and expand into our galaxy, but we already had a lecture on
cosmic sociology (lecture 110 on 2022/5/29) so I'll skip that for today.